Grade Level: Primary School, Middle School, and High School
Math Investigation Type: Shape & Space
Task
Some routine problems dealing with area and perimeter evoke unexpected responses. What to measure, how to measure it, and how to anticipate variations in area and perimeter are often difficult. “If we measure the area of rectangles by dividing them into squares, then what shape should we use to measure area for triangles?” Not such a bad question: Why don’t we use “triangular units” to measure triangular areas?
Part 1
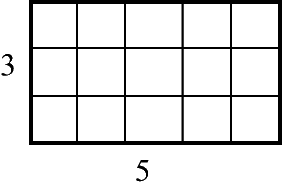
A 3 x 5 index card has an area of 15 square units. You can see that the shape of this card is rectangular. If you want to create a square card with exactly the same area, 15 square units, the dimensions of the side will change. What this means is that the card has the same area as a square that also has 15 square units. It should be possible to cut up the 3 x 5 card into (several) pieces in such a way that these pieces can be put together to form a square card.

There are two problems now:
- How do you construct a square whose sides are the square root of 15, given only a 3 x 5 card?
- The card allows you to measure 3 units and 5 units easily. Put two cards together and 2 units can be measured off quite easily. But how do you measure the square root of 15 units?
- More generally, how do you measure the square root of (x) units, where x can be anything?
- Still more generally, what numbers can be ‘constructed’? For instance, can you draw a line that is pi units long? Or a cube root? Now that you have made the square, how do you cut up the 3 x 5 card so that the pieces can be re-assembled to make the square? What are the minimum number of cuts that you need to make?
Part 2
- Now what if the card isn’t 3 x 5, but some other dimensions. Like 7 x 9. Then how do you cut up the card so that the pieces form a square?
- What’s the general recipe for cutting up a rectangle to create a square?
Part 3
- What is the recipe for cutting up a triangle to make a square?
- Create two squares S1 and S2. How do you cut up S1 and S2 to make a square whose area is the sum of the original areas? What does this have to do with the Theorem of Pythagoras?
Part 4
- Now the big problem…take a convex polygon, how do you cut up the polygon so that when you put the pieces back together you get a square?
