Grade Level: Primary School, Middle School, and High School
Math Investigation Type: Shape & Space
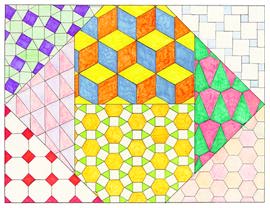
Determining what shapes tile a plane is not a simple matter. There are some polygons that will tile a plane and other polygons that will not tile a plane. For shapes to tile the plane edge to edge without gaps or overlaps, their angles, when arranged around a point, must have measures that add to exactly 360 degrees. If the sum were less, there would be a gap. If the sum were more, the shapes would overlap.
Task
Part 1
- Investigate what shapes can be used to tile a plane. As you work on this portion, post your tentative conjectures and emerging insights to the group so that you benefit from the insights and guidance of your mathematician mentor and the rest of your classmates.
- Test your conjectures by creating a tiling. You can accomplish this in many ways. Make sure that you continue to bring your work and discussions to the rest of the members in your virtual classroom.
- Simple constructed colored drawings work well.
- Or you may choose to use colored construction paper, in which case you would need to carefully construct the shapes, cut them out,and glue them onto another surface.
- Or you may choose to use a computer software program such as Geometer’s Sketchpad by Key Curriculum Press, GeoNext (The GNU General Public License), Cinderella or PlaneTiling Mathematica Package, by Xah Lee.
- What have you learned about what shapes tile a plane?

Part 2
For the second part of this investigation you have a few choices. Choose to do 1 or all of the following tasks:
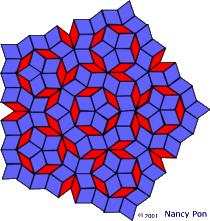
- create Penrose tilings
- create tilings of a sphere (completely)
- create tilings of a representation of a torus. For the torus, I would recommend using a rectangular piece of very flexible cardboard and gluing only with a touch of glue at the center of the tiles. Then, verify that the tiling wraps by bending the cardboard into a cylinder both ways.