Galileo Educational Network – Inspiring a Passionate Commitment to Learning
Category: Number Concepts, Transformations, Patterns, 3D Objects & 2D Shapes, and Measurements
Suitable for Grade Level: Elementary and Secondary
The Math in this Problem:
In this investigation, students are introduced to shapes and designs used within housing designs and blueprints. Applying the idea of convexity, which helps with heat conservation, students will experiment with constructing various complexes using 7 to 12 sides.
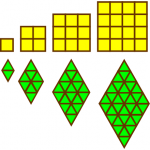
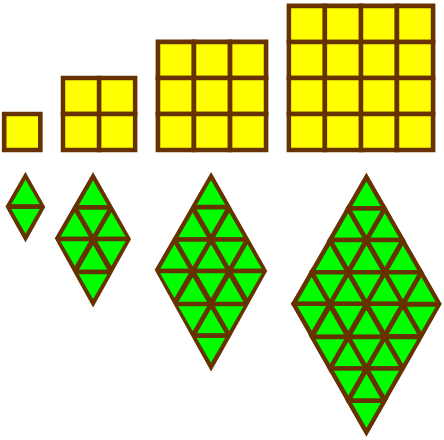
A future development includes the creation of large housing complexes based on two inter-connecting modular units with the same side length:
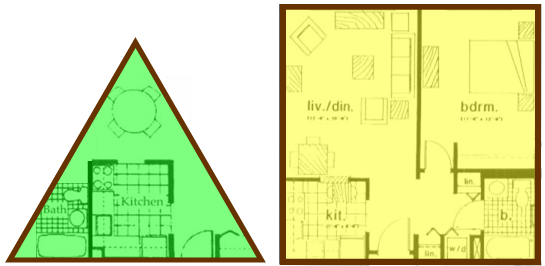
In cold climates, energy conservation demands that we conserve heat. One way to do this is to ensure that any complex we build is convex. A convex complex has the property that if you tie a rope tightly around it, that the rope will always touch the walls.
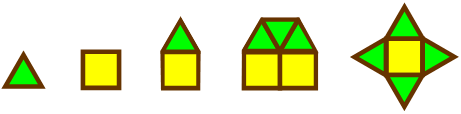
Design convex complexes with 7, 8, 9, 10, 11 or 12 sides.
Extensions:
- Are the complexes you created the only ones possible? Can you prove that there are an infinite number of N-sided solutions for N = 3 to 12?
- Do all the 4-sided complexes differ only in size, but have the same shape? No, there are two 4-sided shapes, a square and a rhombus:
- How many different shapes of complexes are there that are N-sided?
- Is it possible to create a 12-sided complex that is regular (equal angles and sides).
- The square and equilateral triangle produced solutions for N-sided polygons for N = 3 to 12. Find an additional shape that you can add on to your 12-sided complex that allows complexes to be created over N = 13 to 24.
- Find a single modular unit that can be used to create convex complexes with N-sides where N = 3 to 25.