Quinton the Quick Quilter

The Queen was upset. During her gold-hilted sword practice she had missed the target and ended up impaling her favourite quilt to the wall. Although the sword was easily pulled out of the wall, the quilt was left with an unseemly hole. A queen cannot have a hole in her favourite quilt, so she sent forth her royal heralds with instructions to scour her dominions for the greatest quilter to mend her quilt.

The queen advertised a quilting competition to decide who the greatest quilter was. “The winner will be rewarded with gold, jewels, a couple of sheep, a goat, and leftovers from the Queen’s table”. In order to ensure that only the top candidates applied, the queen cleverly added “But those who lose will be thrown to the lions”.

Young maidens with agile fingers and young men with dexterous needlework came from far and wide. They entered the golden palace and were led through it to where the Queen sat on her ancient throne and pointed to a pile of fabric at one end of a coliseum: “Quilters – go to that pile of fabric and make me a square quilt made of exactly 14 smaller squares with no two squares overlapping”. It looked easy enough, but then the Queen said: “To make sure that you work quickly, two lions will now be released from the other end of the coliseum” and with that she gave a flick of her wrist and at the far end of the coliseum, the quilters saw a gate open and two lions enter.
The young maidens with their agile fingers and the young men with their dexterous needlework worked as quickly as they could, but over the few weeks of competition the lions just got fatter.

Some of the square quilts were beautiful, but none of them used exactly 14 squares of fabric. This one is made of 7 squares.

Word of the Queen’s cruel competition spread throughout the land and she was about to think nobody would ever win the competition and that her quilt would never be fixed, when a mere boy showed up at the palace gates and announced that he was Quinton the Quick Quilter. He looked too skinny to be much of a meal for the lions, but she relented, brought him to the coliseum, pointed to the pile of fabric, and told him to make a square quilt of 14 squares just as the fat lions entered the far side of the coliseum.

Now Quinton wasn’t really a Quick Quilter. He hadn’t even tried quilting, but he liked a good challenge. He had timed his visit just right… the lions’ stomachs were scrapping the ground as they slowly made their way down the coliseum. Quinton didn’t wait. He peed around the pile of fabric to disguise his scent and then jumped into it to hide from the lions. From the centre of the fabric pile he could see the lions, but soon they just fell asleep in the corner. The Queen became bored… This was not as exciting as the other quilters. Quinton meanwhile solved the 14 square problem (can you?) and slowly began making a Quilt from underneath his pile of fabric.

The Queen became so angry at this waste of her precious time that she decided to personally take care of Quinton. She grabbed her gold-hilted sword, entered the arena and quietly strode past the sleeping lions towards the pile of fabric hiding Quinton. Quinton yelled as loud as he could. The lions awoke. The Queen fought valiantly, but the two lions were too much. After the royal meal, the lions went back to sleep again and Quinton wrapped himself in his Quilt, took the gold-hilted sword, and pronounced himself King.

Extensions:

• Is it possible to make a square quilt made up of 2 squares? 3 squares? What are all the numbers that are impossible?
• Design a similar problem for triangles.
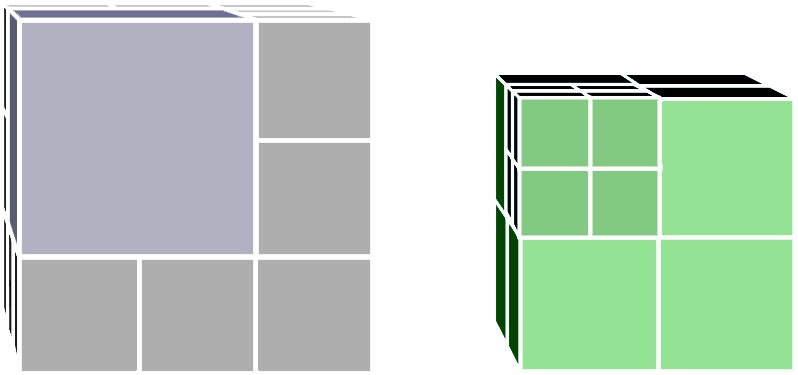
• How many cubes are each of the below cubes created from? A cube cannot be made of 47 smaller cubes, but any larger number is possible. What numbers below 47 are possible?

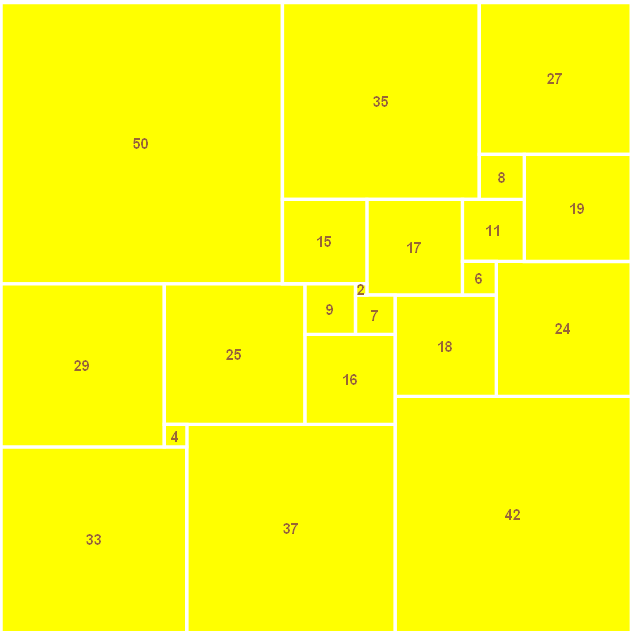
• It was once thought to be impossible to create a square using all differently sized squares. Then the following square was discovered where the number in each square is its side length. Create some puzzle based on this square.