Menu Close

Ring of Threes

Download PDF

Category: Number Concepts, Number Operations, and Variables & Equations
Suitable for Grade Level: Elementary and Secondary

The Math in this Problem:

Ring of Threes is a math investigation involving the application of multiplication and addition with single digits. Students will have to analyze various number sequences, while applying these basic mathematical operations.

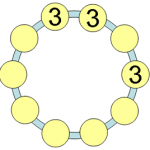
Write 1’s and 2’s in the circles so that no three consecutive numbers sum to a multiple of three.

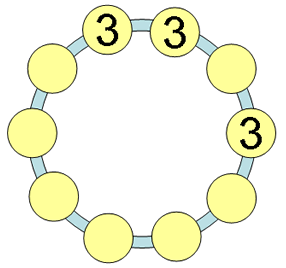
Extensions:

  • There are four solutions to the original problem with 10 circles. Can you find them all? Create your own problem by repositioning the 3s. Is it always possible to solve? Is it possible to create a problem with exactly one solution?
  • Is it possible to place the numbers 1 to 8 in the circles below so that the following rules are obeyed?
    • no 2 consecutive numbers sum to a multiple of 2.
    • no 3 consecutive numbers sum to a multiple of 3.
    • no 4 consecutive numbers sum to a multiple of 4.
    • no 5 consecutive numbers sum to a multiple of 5.
    • no 6 consecutive numbers sum to a multiple of 6.
    • no 7 consecutive numbers sum to a multiple of 7.
    • no 8 consecutive numbers sum to a multiple of 8.
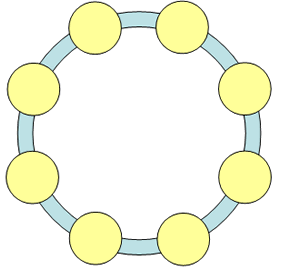
  • If this is impossible, can at least some of the rules be obeyed?
  • Is it possible to place the numbers 1 to 9 in the circles below so that the following rules are obeyed?
    • no 2 consecutive numbers sum to a multiple of 2.
    • no 3 consecutive numbers sum to a multiple of 3.
    • no 4 consecutive numbers sum to a multiple of 4.
    • no 5 consecutive numbers sum to a multiple of 5.
    • no 6 consecutive numbers sum to a multiple of 6.
    • no 7 consecutive numbers sum to a multiple of 7.
    • no 8 consecutive numbers sum to a multiple of 8.
    • no 9 consecutive numbers sum to a multiple of 9.