The Cube of Many Colours

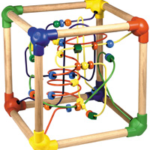
Annie Ant works hard all day, carrying dead insects back to her Ant Hill to help feed her thousands of brothers and sisters. At the end of a hard day of work, there is nothing Annie likes to do better than to go exploring. One day she crawled up to a human house, squeezed under the door and tip-toed her way into a small human’s bedroom. There, in the middle of the floor, lay an object that radiated a magical energy:

Surely, Annie thought, this cube-of-many-colours is where rainbows play when they are not jumping around outside.

Annie was a brave ant, but even she was too scared to touch the cube-of-many-colours*. She decided to go back to her ant hill and bring her brothers and sisters to see the cube-of-many-colours. When she asked Egor Ant, he said he was too busy making termite stew. When she asked Lizzy Ant, she said she was too busy cleaning her antenna. When she asked Willy Ant, he said he was too busy carrying pupae to the new nursery. And so it was with all the ants – they all were too busy to go and see Annie Ant’s cube-of-many-colours.

So Annie Ant decided to go back by herself… and this time she was going to be brave. She squeezed under the baby’s door, saw the cube in the middle of the floor, crawled near to it, gulped, and then jumped onto her favourite yellow corner. “I am very brave” she thought to herself.

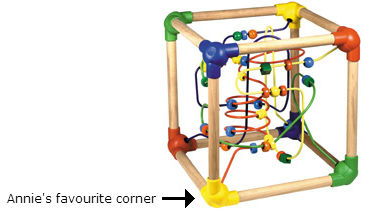
Only using the wooden struts between corners, Annie visited all 8 corners of the cube-of-many-colours and returned to the same yellow corner that she started from without using a wooden strut more than once. “Yippee!” She thought – “I am a brilliant ant.”

Annie took four cards out of her pocket. “I am going to see how long it takes me to move to the opposite corner if I move randomly across the wooden struts” she said.

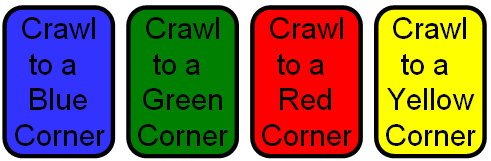
Since she was on her favourite, yellow corner, she put the yellow card away and then randomly chose one of the remaining three colours. She chose the blue so she climbed till she was at the blue corner. Then she took the yellow, red and green cards and randomly chose her next corner… she continued this until, after 5 moves she arrived at the yellow corner at the far side of the cube. “That was interesting” thought Annie Ant, “I did that without visiting a green corner”. I’m going to repeat my experiment ten times and see if I notice any patterns.

Annie Ant did find some patterns. Can you?

Extensions:

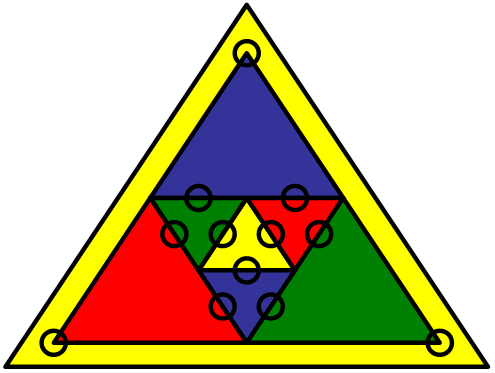
• One day when Annie was randomly wondering around the cube-of-many-colours she thought of Grandma Ant’s triangular house. Maybe this cube is like Grandma’s house except that the wooden struts on the cube are replaced by wooden revolving doors (circles). What do you think?

• Another day, Annie Ant convinced her mother’s sister (who had retired) to visit the cube-of-many-colours. Annie had forgotten to make an extra set of cards, so they started on opposite yellow corners of the cube-of-many-colours and then Annie drew a card. It was blue, so Annie moved up from her favourite yellow corner and her mother’s sister moved down. Annie said “Let’s play till we meet on the same corner – then let’s go and eat.” How long do you think they drew cards before they ate?
• One night, Annie dreamt a strange dream. She was walking around her cube-of-many-colours – starting on her favourite yellow corner and trying to crawl on all of the wooden struts, without using any of them more than once. It didn’t work. “The cube doesn’t allow me to do that” Annie thought. “I wonder why?” Can you help Annie Ant find the reason why the cube doesn’t allow her to do that?
• One day Annie ant returned to find that her cube-of-many-colours was replaced by something called a tinker-cube. All the colours had vanished from the corners and Annie Ant was sad. But then she smiled, took out her 4 cards again, and started wandering around randomly just like before. Can you find a different tinker-cube that Annie ant could play on?

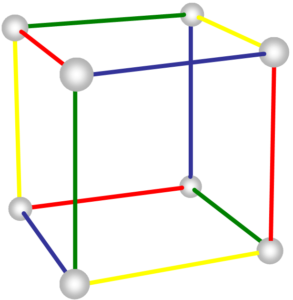
• Prove that the number of steps taken by Annie Ant in going from one corner of a cube to the opposite corner is always odd. The following diagram may help: