Menu Close

Injection Molding

Download PDF

Category: 3D Objects & 2D Shapes, Measurements, Number Operations, Transformations, and Patterns
Suitable for Grade Level: Elementary and Secondary

The Math in this Problem:

Injection molding is a technique for constructing plastic objects, including toothbrushes, hair combs, buttons, and food containers. This math puzzle introduces students to the concept of injection molding and its relationship with mathematics, as it pertains to the complex geometry of creating various products with different shapes and sizes.

Injection molding is a technique for making a huge range of plastic objects like toothbrushes, fly swatters, and food containers. Molten hot plastic is injected into a mold at high pressure. When the plastic cools, it solidifies and can be removed from the mold.

The molten hot plastic is injected into the mold, at one or more injection sites. The further away a part of the mold is from an injection site, the more difficult it is for the molten plastic to get there.

The molten plastic cannot flow through the fly swatter holes, so distance must be calculated by moving horizontally and vertically. If a single injection site (the red dot) is used for the head of the fly swatter the molten plastic must travel 12 cm to get to all the corners (see above). A good choice for a set of injection points doesn’t allow any point to get too far away from an injection site.

  • Position 2 injection points so that the molten plastic only has to travel 9 cm.
  • Position 3 injection points so that the molten plastic only has to travel 8 cm.
  • Position 4 injection points so that the molten plastic only has to travel 6 cm.
  • Position 13 injection points so that the molten plastic only has to travel 3 cm.
One Solution
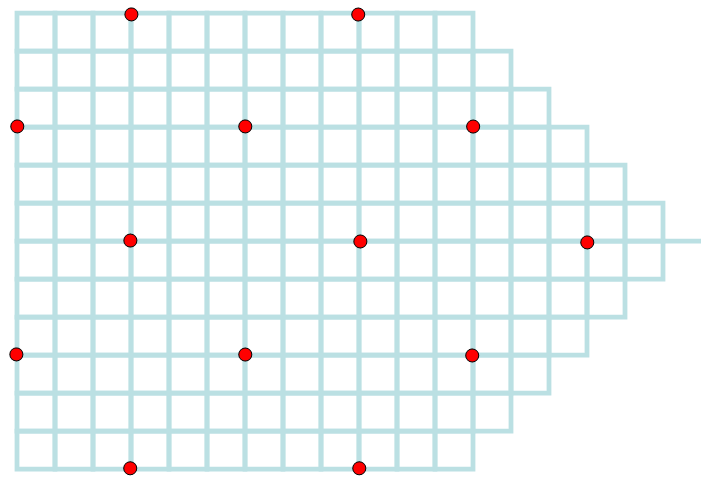
Above is a good solution for 13 injection points which only requires the molten plastic to flow 3 cm.

  • Position 27 injection points so that the molten plastic only has to travel 2 cm.

Extensions:

Thousands of these blue & green plastic name tags were ordered by a tour bus operator:

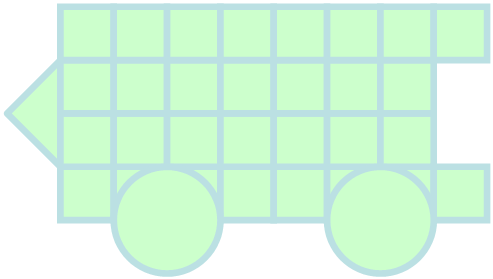
If you use a single injection point (shown in red below), some of the molten plastic must ooze more than 5cm. Why is the point marked with an arrow labeled √13+1 instead of √20?

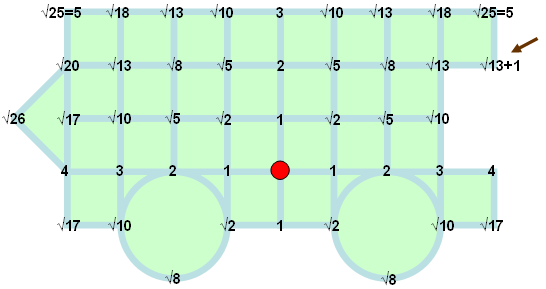
Questions:

  • Choose two injection points on the plastic name tag so that any point is at most 3 away from an injection point.
  • How many injection points do you have to use so that any point is at most 2 away from an injection point?
  • Under some conditions the injection molding problem is equivalent to finding the smallest number of circles that can cover the object. What are these conditions?
  • Lost wax bronze sculpting uses a similar idea to injection molding. You can see a video of this process here. How would extend the injection molding problem to three dimensions.